Special relativity and the rod/slot paradox – IX – transit scenario and measurements by an observer co-moving with the rod

In the course of this series on the rod/slot paradox in Special Relativity we now start to analyze a scenario in which a rod moves through a slot. We have called such a scenario a transit scenario. I want to show that an inclination of the slot against the x-axis (and the rod) measured in a frame co-moving with the rod removes any potential paradox. To achieve this objective we apply a Lorentz Transformation [LT] to get coordinates of key events as measured by an observer co-moving with the rod.

The readers who have followed my line of argumentation for proving consistency between the descriptions of a rod/slot collision in various inertial frames have certainly got an idea already, of what is going to happen. Our intuition tells us that a systematic time shift of distanced events measured by moving observers will cause an inclination angle of the slot versus the x-axis (and thus versus the slot, too) in a frame co-moving with the rod. The additional diagonal movement of the slot vs. the rod in the rod’s frame of reference may help to guarantee a collision-free rod-slot encounter.

The transit scenario

For the setup of our transit scenario see the previous 8th post in this series. In a frame Z, a plate with a slot moves vertically upwards along the y_z-axis with constant velocity v_y. At time t_Z = 0 the slot’s right end meets the right end of a rod moving horizontally with constant velocity ν_x along the line y_Z = 0. Slot and rod both have a proper length of L. The situation at t_Z = 0 is schematically depicted in illustration 1:

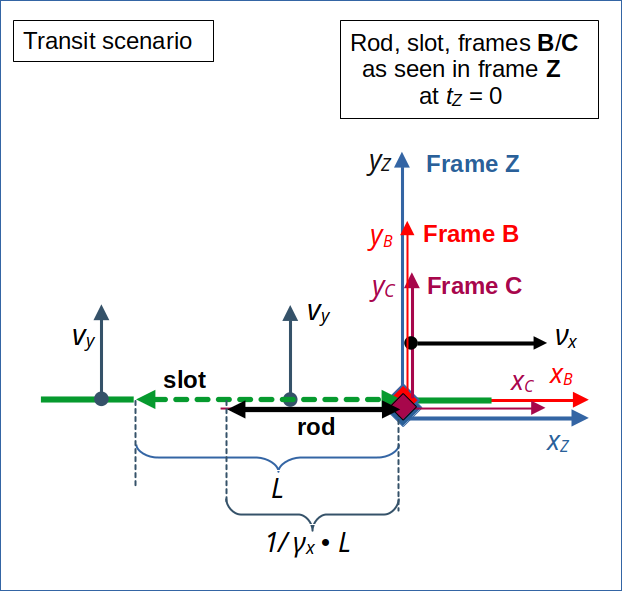
Transition scenario: At t = 0 the rod's right end meets the slot's right end

Illustration 1: The right end of a vertically upward moving slot meets the right end of a horizontally moving rod as seen in a frame Z at the origin x_Z = 0 at time t_Z = 0. The rod and its attached reference frame C move to the right in x-direction with a (positive) constant velocity ν_x. The slot, instead, moves upwards with constant velocity v_y. At t_Z = t_C = t_B = 0, the origins of all the depicted frames’ spatial coordinate systems coincide.

We use Cartesian coordinate systems to describe the 2-dimensional object movements in a globally flat Euclidean space.

Frames B and C are inertial systems co-moving with the slot and the rod, respectively. Due to the relativistic length contraction effect an observer in frame Z predicts a collision-free transit of the rod through the slot or, equivalently, a passage of the slot across the rod. The transit happens at the very last possible moment for our infinitesimally thin objects.

Lorentz Transformation of key event coordinates to Frame C co-moving with the rod

To find out what an observer in frame C measures, we closely follow the recipes we have successfully applied for the collision scenario in the previous posts of this series. We apply the Lorentz Transformation formulas (see the 3rd post in this series) to space and time coordinates describing the location of the slot’s end in frame Z. Our objective is to get corresponding coordinates in frame C.

Note: We select events in frame Z such that the transformation of the respective time coordinates results in one and the same time coordinate t_C = 0 in frame C. Readers of my previous posts know already how to determine respective events in Z. I just define them below.

Coordinates get subscripts to relate them to our objects: The first subscript is “s” or “r” for the slot or rod, respectively. The second one is “r” or “l” for the objects’ left or right ends, respectively. The last index is “Z”, “B”, or “C” – indicating the frame where the coordinate is measured. We will use the following standard definition of basic relativistic factors occurring in the LT formulas:

\[ \begin{align} \beta_x &= {\nu_x \over c} \lt 1 \,, \quad \gamma_x = {1 \over \sqrt{ 1 – { \nu_x^2 / c^2}\, }} \gt 1 \,, \\[10pt] \beta_y &= {v_y \over c} \lt 1 \,, \quad \gamma_y = {1 \over \sqrt{ 1 – { v_y^2 / c^2}\, }} \gt 1 \,. \end{align} \]

We use the following events and calculate their coordinates in frame C :

E1 for the slot’s right end:

\[ \begin{align} \pmb{ \operatorname{E1}}: x_{s,r,Z}|_{E1} &= 0 \quad \Rightarrow \\ x_{s,r,C}|_{E1} &= \gamma_x \,(0 \,-\, \nu_x * 0) \,=\, 0, \\[10pt] y_{s,r,Z}|_{E1} &= 0 \quad \Rightarrow \\ y_{s,r,C}|_{E1} &= 0 \,, \\[10pt] t_{s,r,Z}|_{E1} &= 0 \quad \Rightarrow \\ t_{s,r,C}|_{E1} &= \gamma_x \,( 0 \,-\, \nu_x/c^2 * 0) \,=\ 0 \,. \end{align}\]

E2 for the slot’s left end:

\[ \begin{align} \pmb{ \operatorname{E2}}: x_{s,l,Z}|_{E2} &= \,- L \quad \Rightarrow \\ x_{s,l,C}|_{E2} &= \gamma_x \,\left( – L \,-\, \nu_x * (- {\nu_x \over c^2}) \right) \,= \, \,- {1 \over \gamma_x} * L, \\[10pt] y_{s,l,Z}|_{E2} &= \,- {\nu_x * v_y \over c^2} \, L \quad \Rightarrow \\ y_{s,l,C}|_{E2} &= \, – {\nu_x * v_y \over c^2} \, L\,, \\[10pt] t_{s,l,Z}|_{E2} &= \, – {\nu_x \over c^2} * L \quad \Rightarrow \\ t_{s,l,C}|_{E2} &= \gamma_x \,\left( – {\nu_x \over c^2} \, L \,-\, \nu_x/c^2 * ( – {\nu_x \over c^2} \, L) \right) \,=\, 0\,. \end{align}\]

The coordinate y_s,l,Z < 0 results from the fact that we have to choose a time coordinate t_s,l,C < 0 to get spatial coordinates at t_C = 0 for E2.

Using our short notation for events (see previous posts) this means:

\[ \begin{eqnarray*} &\pmb{ \operatorname{E1}}:\,\, &[\,0,\,&0,\,&0&\,]_{E1,Z} &\,\, \Rightarrow \,\, &[\,0,\,&0,\,&0 &\,]_{E1,C}, \\ &\pmb{ \operatorname{E2}}:\,\, &[\,- L,\, &\,- {\nu_x * v_y \over c^2} \, L , &\, – {\nu_x \over c^2} \, L&\,]_{E2,Z} &\,\, \Rightarrow \,\, &[\,\, – {1 \over \gamma_x} \,L,\, &\,- {\nu_x * v_y \over c^2} \, L,\, &0 &\, ]_{E2,C} \end{eqnarray*}\]

We get something similar for events E3 (right end of rod) and E4 (left end of rod).

E3 for the rod’s right end:

\[ \begin{align} \pmb{ \operatorname{E3}}: x_{r,r,Z}|_{E1} &= 0 \quad \Rightarrow \\ x_{r,r,C}|_{E1} &= \gamma_x \,(0 \,-\, \nu_x * 0) \,=\, 0, \\[10pt] y_{r,r,Z}|_{E1} &= 0 \quad \Rightarrow \\ y_{r,r,C}|_{E1} &= 0 \,, \\[10pt] t_{r,r,Z}|_{E1} &= 0 \quad \Rightarrow \\ t_{r,r,C}|_{E1} &= \gamma_x \,( 0 \,-\, \nu_x/c^2 * 0) \,=\ 0 \,. \end{align}\]

Regarding the left end of the rod, we have to be careful. The LT tells us that we need to pick a shifted point t_r,l,Z (< 0 ) in time, according to

\[ \begin{align} t_{r,l,C}|_{E4} \,= \, \left(\, t_{r,l,Z}|_{E4}\,\, – \, {\nu_x \over c^2} * x_{r,l,Z}|_{E4} \, \right) \, = \, 0 \,. \end{align} \]

to get a corresponding t_r,l,C= 0. But because the rod is moving horizontally in frame Z, the left end of the rod resides at

\[ x_{r,l,Z}|_{E4}\,= \, – \,{1 \over \gamma_x} L \,+ \, \nu_x * t_{r,l,Z}|_{E4} \]

at this point in time (t_r,l,Z < 0). This in turn means

\[ \begin{align} &t_{r,l,C}|_{E4} = 0 \quad \Rightarrow \\[8pt] &t_{r,l,Z}|_{E4} \, – \, {\nu_x \over c^2} \left( – {1 \over \gamma_x} L + \nu_x * t_{r,l,Z}|_{E4} \right) = 0 \quad \Rightarrow \\[8pt] &t_{r,l,Z}|_{E4} \,=\, – {\nu_x \over c^2} \gamma_x\,L \,. \end{align} \]

Thus

\[ \begin{align} x_{r,l,Z}|_{E4} \,&=\, – {1 \over \gamma_x} L + \nu_x \left( – {\nu_x \over c^2} \gamma_x \,L \right) \\[8pt] &=\, – {1 \over \gamma_x} \, L * \left( 1 \,+\, \gamma_x * {\nu_x^2 \over c^2} \right) \\[8pt] &= \, -\gamma_x * L \,. \end{align} \]

The last equation follows from evaluating γ_x * β_x. We get the following results for the space and time coordinates :

E4 for the rod’s left end:

\[ \begin{align} \pmb{ \operatorname{E4}}: x_{r,l,Z}|_{E4} \, &= \,- \gamma_x * L \quad \Rightarrow \\ x_{r,l,C}|_{E4} \, &= \gamma_x \,\left( \, – \gamma_x * L \,-\, \nu_x * ( \,- {\nu_x \over c^2} * \gamma_x * L ) \,\right) \\ & = \, – L * \left( \gamma_x^2 \,-\, \gamma_x^2 * {\nu_x \over c^2 } \right) \\ & = \, – L \,, \\[10pt] y_{r,l,Z}|_{E4} \, &= 0 \quad \Rightarrow \\ y_{r,l,C}|_{E4} \, &= 0\,, \\[10pt] t_{r,l,Z}|_{E4} \, &= \, – {\nu_x \over c^2} * \gamma_x * L \quad \Rightarrow \\ t_{r,l,C}|_{E4} \, &= \gamma_x * \left( – {\nu_x \over c^2} * \gamma_x * L \, – \, { \nu_x \over c^2} * ( – \gamma_x *L ) \right) \\ &=\, 0\,. \end{align}\]

This is exactly what we expect for the rod due to our setup!

\[ \begin{eqnarray*} &\pmb{ \operatorname{E3}}:\,\, &[\,0,\,&0,\,&0\,]_{E3,Z} &\,\, \Rightarrow \,\, &[\,0,\,&0,\,&0 \,]_{E3,C}, \\ &\pmb{ \operatorname{E4}}:\,\, &[\,-\gamma_x * L,\,&- {\nu_x \over c^2} * \gamma_x * L,\,&0\,]_{E4,Z} &\,\, \Rightarrow \,\, &[\,- L,\, &0,\, &0\, ]_{E4,C} \end{eqnarray*}\]

Regarding the transformation of velocities, the LT gives us a reduced vertical velocity of the rod in frame C due to time dilation :

\[ v_{y,C} \,=\, {1 \over \gamma_x} * v_y \,. \]

Let us find a geometrical interpretation of our results …

Inclination angle between the rod and the slot in frame C

The data above support the following perception of an observer in frame C at t_C = 0:

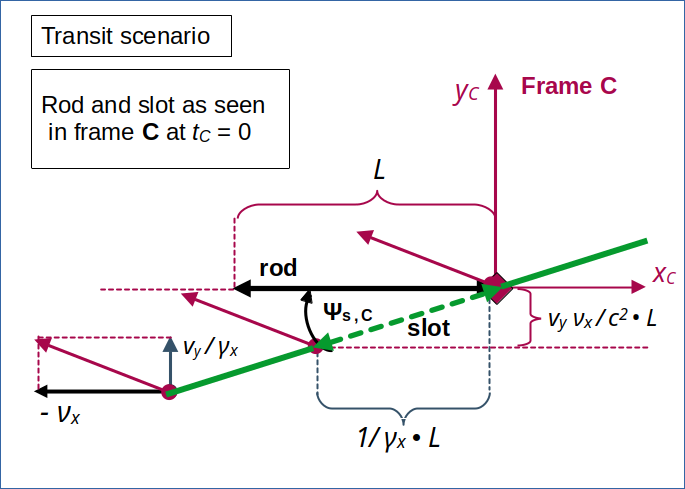
transit scenario from the perspective of the rod in frame C

Illustration 2: Perception of the slot passing the rod at t_C = 0 in frame C – without collision! The inclination angle Ψ_s,C and the diagonal movement of the slot guarantee a smooth transit of the rod.

This gives us an idea of how the slot could pass the rod without a collision. The resulting inclination angle Ψ_s,C helps the slot to pass the rod in diagonal direction: If the resulting diagonal velocity of the slot in frame C moved the left end of the slot to a point to the left of the rod on line y_C = 0, then the surrounding plate would not touch the rod. We will look at the encounter and transit of the rod in more detail in the next post.

Just to feel a bit safer, let us find out, when the the left end of the slot reaches the line y_C = 0. The time to overcome the vertical distance, which the left end of the slot must pass to reach y_C = 0, is given by :

\[ \begin{align} \Delta t_y \,&= \, {\nu_x * v_y \over c^2} * L * \gamma_x * {1 \over v_y} \\ &=\, \gamma_x \, {\nu_x \over c^2} * L \,. \end{align} \]

During this time the left end of the slot moves to the following point left on the x_C-axis:

\[ \begin{align} x_{s,C}\left(t_C = \gamma_x \, {\nu_x \over c^2} * L \right) \, &= \, – {1\over \gamma_x}*L \,- \, \nu_x * \Delta t_y \\[8pt] &= \, – {1\over \gamma_x} * L \,-\, \nu_x * \gamma_x \, {\nu_x \over c^2} * L \\[8pt] &=\, – L * \left( {1 \over \gamma_x} \,+\, \gamma_x \,{\nu_x^2 \over c^2} \right) \\[8pt] &=\, – \gamma_x * L *\left( \beta_x^2 + {1 \over \gamma_x^2 } \right) \\[8pt] &=\, – \gamma_x * L \,\gt\, L\,. \end{align} \]

This result, indeed, guarantees a collision free passage of the slot.

Conclusion

In this post we have analyzed what an observer co-moving with the rod would experience at t_C = 0 in our “transit scenario”. Such an observer would measure the slot not only to move diagonally, but also to have a permanent inclination angle Ψ_s,C with the x_C-axis and the rod. Combining the diagonal movement with the slot’s inclination appears to allow for a collision free meeting of the slot and the rod. The rod just moves through the slot, while the slot passes in diagonal direction.

We will analyze the geometry of the transit process in more detail in the next post.

Special relativity and the rod/slot paradox – X – details of the transit scenario as seen by the rod

For the time being we can safely assume that both an observer in our setup frame Z and an observer in a frame C co-moving with the rod would predict a collision-free transit of the rod through the slot. Obviously, the Lorentz Transformation once again helps to guarantee consistent descriptions by observers located in different inertial frames.

Stay tuned …